Mil-Dot Made Easy

Editor’s Note: All rifle shooters should have a grasp of either mils, MOA, or both. A “grasp” is required to even properly adjust a scope for zeroing. If you want to be a great rifle shooter you’ll master the concepts.

The Mil-Dot system is exceptionally versatile – if you know how to use it. If you drive a submarine, you can use them to figure out how far away you are from a ship that needs torpedoing. If you shoot a rifle, you can use mil-dot to hold the correct amount over a distant target. Or, if you have the system on an optic like a spotting scope, rifle scope or binoculars, you can use it to accurately figure out how far away something (or someone) is.

There’s a lot of fancy math behind the whole mil-dot thing, but we’re going to ignore most of that here and explain the concept, and more importantly, how you can use it, in plain English. There won’t even be a quiz at the end, I promise.

The Math

The biggest problem with mil-dots is that most people try to explain them using big words like tangent, subtension, and fractions. We’ll try to avoid that here. I’m going to break my promise about not talking about math, but only for a second, so hold your nose and read on. I’ll make it painless, really.

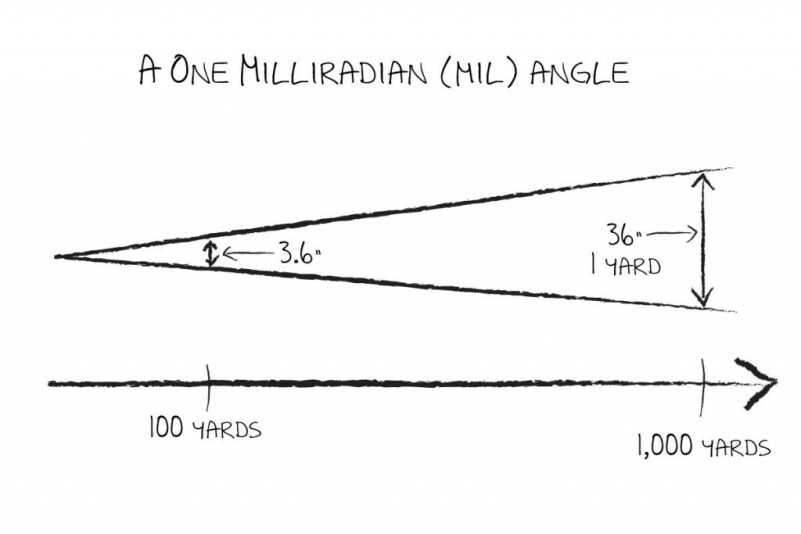
Mil-dot is an abbreviation for “milliradian” and “dot”, which is from the English word meaning, dot. A milliradian is 1/1000th of a radian. What’s a radian? It’s a measure of distance traveled around a circle. So if you start eating the crust of a whole double-cheese and sausage pizza and work your way exactly half way around, you’ll have eaten 3.14159 radians of pizza crust. That’s half a circle, or three slices, as I like mine cut. It doesn’t matter how big the pizza is because radians measure the amount of travel around a circle of any size. Radians basically measure an angle. If you think of the same amount of munched pizza crust in terms of degrees, those 3.14159 radians are the same as 180 degrees.

Recognize that 3.14159 number? Yep, that’s Pi. Amazing coincidence that I used both Pi and pizza in this example, isn’t it? If you remember junior high math, Pi helps measure circles. Half the diameter of a circle, times two, times Pi, equals the distance all the way around. So, there are 6.28 radians in any circle. Since there are 1000 milliradians in a radian, a circle is comprised of 6,280 milliradians.

Just because!

Here’s where people who really hate math make things a bit confusing. The U.S. Army says that there are 6,400 milliradians in a circle, not 6,280. Just because. It’s like arguing with your little brother. You can end debate by saying “Because!” with lots of conviction and volume. You know the Army has lot’s of conviction and even more volume. The Russians certainly aren’t going to agree with the U.S. Army, so they say there are 6,000 mils in a circle. Again, just because. We won’t get into a debate here of whether “because” from the Americans wins over “because” from the Russians. So, for this reason of “just because” from here on out we’re going to go with the theory that there are 6,400 mils (milliradians) in a circle. Just because.

What exactly is a milliradian?

So what’s a milliradian? It’s a pretty small angle in practical terms. If you stand at the shooting bench and hold your arms out in a really, really small angle of exactly one milliradian and looked between your outstretched hands, you would only be able to see 3.6 inches of your target if it was 100 yards away. Since angles work proportionally, if you looked at something 200 yards away, you would see 7.2 inches. If you looked at an object 1,000 yards away, you would see 36 inches of your target. See how that works? The angle of a milliradian translates to 1 yard at 1,000 yards range or just 3.6 inches at 100 yards range.

Mils and rifles

When you shoot a rifle exactly parallel to the ground, the bullet starts to fall immediately. Because gravity. This applies to both the U.S. Army, the Russians, and all the rest of us. When people talk about a bullet “rising” at first, that’s only because the barrel is pointed slightly upward from exact parallel due to the arrangement of the scope to the rifle barrel.

So, if you’re trying to hit a target 100 yards away, you have to aim a little “up” and if you’re trying to hit a target 1,000 yards away, you have to aim a lot “up.” You could work all this out in inches, meaning you would figure out you need to aim maybe 1.5 inches high for the 100-yard target and something like 400 inches high for a target 1,000 yards downrange.

The problem with using inches is that you can’t create scope adjustment dials with all those numbers marked in inches. If each click of your scope corresponded to an inch, what distance would that work for? Maybe 100 yards? What if you’re shooting at a target 367 yards away? Then how many inch-clicks do you turn? Imagine your spotter has figured out you need to aim 13.7 inches high to hit a target 441 yards away. When he says “adjust up 13.7 inches!” you’re like… what? How many clicks is that?

This is one of the reasons why we use milliradians to standardize the amount of adjustment we’re going to make. If we need to aim 3.6 inches high for a 100-yard target, we make a one milliradian adjustment. If we need to aim 7.2 inches high to hit a target 200 yards away, we make a one milliradian adjustment. See how that works? Now, your spotter can just tell you something like “add one mil of elevation.”

Just so you know I didn’t forget, all this applies to windage (sideways) measurement too, it’s just easier to use elevation, or bullet drop, examples. An angle is an angle, regardless of whether it measures something up and down or sideways.

How to figure it out

Next, we need a way to calculate inches at a certain distance to the standardized mil measurement. If you range a target and figure out that it’s 428 yards downrange, you can use a ballistic program or chart to determine how far your particular bullet will drop at that range. That will depend on your caliber, cartridge, and gun combination. For example’s sake, let’s assume we’re shooting a .308, and we figure the bullet will hit 36 inches low at that distance. How many mils do you adjust your scope elevation to get an accurate hit?

Fortunately, there’s a really easy way to calculate this without using complex math stuff like tangents and cotangents. The number of mils equals the amount of bullet drop (measured in yards) times 1000, divided by the distance to target in yards. In our example, we have 36 inches, or one yard of bullet drop and a range of 428 yards.

Mils = (1 yard * 1,000) / 428 yards = 2.3 mils.

If your scope is measured in 1/10th mil clicks, that’s 23 clicks in upward elevation adjustment. Make sense?

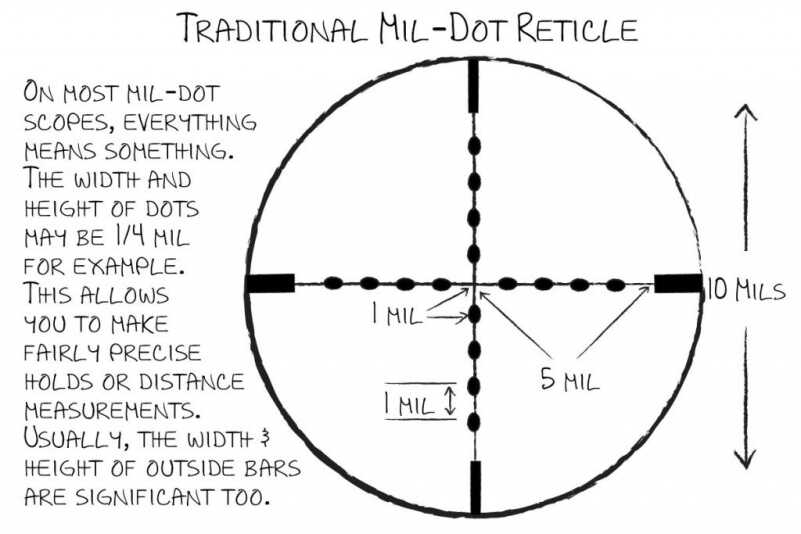
Mil-dot reticles

Mil-dot scope reticles look really cool. They will have normal crosshairs with a series of dots, evenly spaced horizontally and vertically along the crosshairs. The distance from the center of one dot to the center of the next is exactly 1 milliradian, or mil. Most traditional mil-dot scopes have graduations that show 10 mils vertical and 10 horizontal. There is no dot in the exact intersection of the crosshairs as that would be annoying and block your view of the target. Consider the intersection of the crosshairs as the center of a dot, so the distance from that intersection to the center of a dot in any direction is exactly one mil.

In our example, we talked about using turret click adjustments to move the crosshairs to exactly the right place to account for elevation changes, but you can also just use the dots on the reticle. If you have to aim exactly 3 mils high to hit a distant target, you can place the second dot (Correction: “Second dot” was a typo, it’s the third dot!) below the crosshairs on your target to achieve exactly the same thing. It’s a quick and easy way to adjust, although not as precise as making fine click adjustments.

You can use the horizontal mil-dot markings to lead moving targets by the right amount also. I you have to lead a target by 36 inches and it’s 1,000 yards away, then place the first horizontal crosshair dot over the target.

Then what the heck is Minute of Angle?

Some scopes have turrets that make adjustments measured in mils, with .1 mil per click being common. However, many optics use a similar, but different, way to measure angular adjustments called minute of angle, or MOA for short. Your optic might have turrets that move one, ½ or even ¼ minutes of angle for each click.

A minute of angle also represents a slice of a circle, just measured differently. If we sliced our pizza into 360 pieces of equal size, each piece would be a degree as a circle contains 360 degrees total. If you divide each degree, or single slice, into 60 equal parts, those would be called minutes. Since each degree is divided into 60 minutes, a complete circle has 21,600 minutes of angular measurement. With me so far?

Using the same concepts that we used to translate an angle to a number of inches at a certain distance, we can do the same with minutes of angle. Instead of one minute of angle translating to 3.6 inches at 100 yards, it works out to 1.04 inches at 100 yards. A minute of angle is an even narrower measurement than a milliradian.

The basic math process to figure out how many minutes of angle correspond to inches of bullet drop (or windage adjustment) at a given range works like this.

Minutes of Angle = Correction in inches / Hundreds of yards.

So, using our earlier example, if we have to aim 36 inches high to hit a target at 428 yards downrange, then the equation works like this:

MOA = 36 / 4.28 hundreds of yards = 8.41 MOA.

Since MOA’s are “smaller” you need more of them than mils to make the same amount of adjustment. If your scope adjusts in ¼ MOA clicks, then you will raise your desired point of impact 8.41 MOA / .25 MOA per click, or 34 clicks. It’s actually 33.6 clicks, but you can’t do .6 of a click!

Practical application

So all this abstract stuff is great, but what do you do with it?

For starters, use these concepts to zero your rifle at the range. Plenty of folks zero their rifle for 100 yards. Without fail, I see folks with their new rifle at the range firing a shot, spinning the dials randomly, then repeating the process. They’ll spend lots of time and ammo hoping to get on target by trial and error. Here’s how easy it is to use some basic math to get on target quickly.

• Fire a shot or small group at your target, 100 yards down range.
• Measure how many inches you need to move the hits to be exactly where you want them. Suppose your bullets are hitting 3 inches above the bullseye.
• Since you are shooting at 100 yards, you need to move your scope adjustment down 3 inches, which conveniently works out to 3 MOA.
• If your scope has ¼ MOA click adjustments, then turn your dial 12 clicks (¼ per click times 3 MOA) to get on target.

Piece of cake, right?

What’s next?

This article is a basic introduction to the concept of mils and how to convert bullet drop measurements into milliradian adjustments. But mils are much more powerful than that. You can also use mils for range estimation, just by looking at objects of known size through your binoculars, spotting scope, or rifle scope. Next time, we’ll cover that topic.